X-Shells: A New Class of Deployable Beam Structures

Abstract

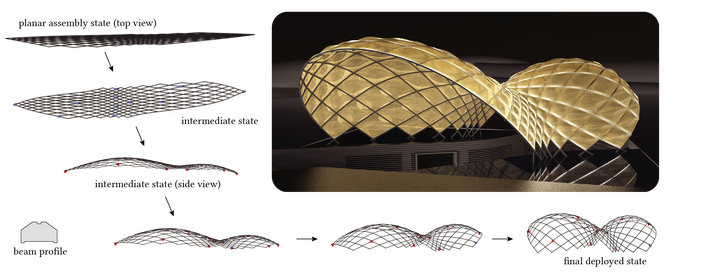
We present X-shells, a new class of deployable structures formed by an ensemble of elastically deforming beams coupled through rotational joints. An X-shell can be assembled conveniently in a flat configuration from standard elastic beam elements and then deployed through force actuation into the desired 3D target state. During deployment, the coupling imposed by the joints will force the beams to twist and buckle out of plane to maintain a state of static equilibrium. This complex interaction of discrete joints and continuously deforming beams allows interesting 3D forms to emerge. Simulating X-shells is challenging, however, due to unstable equilibria at the onset of beam buckling. We propose an optimization-based simulation framework building on a discrete rod model that robustly handles such difficult scenarios by analyzing and appropriately modifying the elastic energy Hessian.

This real-time simulation method forms the basis of a computational design tool for X-shells that enables interactive design space exploration by varying and optimizing design parameters to achieve a specific design intent. We jointly optimize the assembly state and the deployed configuration to ensure the geometric and structural integrity of the deployable X-shell. Once a design is finalized, we also optimize for a sparse distribution of actuation forces to efficiently deploy it from its flat assembly state to its 3D target state. We demonstrate the effectiveness of our design approach with a number of design studies that highlight the richness of the X-shell design space, enabling new forms not possible with existing approaches. We validate our computational model with several physical prototypes that show excellent agreement with the optimized digital models.

Errata

The originally published version of our paper and supplement claimed errors in the derivative formulas reported in [Bergou et al. 2010]. Specifically, we pointed to the omission of terms without which the Hessian and gradient expressions disagree, causing issues in certain situations like finite difference validations. However, the original formulas are valid when used in the specific way the authors intended, which we thank them for clarifying. The updated PDFs linked here clarify these subtleties, derive the additional terms, and comment on in which situations these new terms should be used.